Common Relativity

There’s General Relativity and Special Relativity. And then, there’s the relativity we encounter every day.

I’ve noticed that, when Einstein’s Theory of Relativity is discussed, it’s often described as “surprising” or “complicated”.

While it certainly was surprising at the time it was discovered, and the equations behind it may well be a bit complicated, I think the basic ideas are quite straightforward.

Or, at least, you can get used to them, the same way you get used to the strange way relativity works in day-to-day life.

Einstein had two kinds of relativity, special and general. Contrary to popular belief, Special Relativity is not more special or advanced than General Relativity. It’s just a “special case”. It works only in certain cases, when things are moving smoothly and not changing the speeds or directions in which they go.

Both kinds of relativity show their effects only when very high speeds or gravities are involved. In everyday life, their effects are so small that nobody noticed them for a very long time. Still, there are other, more common kinds of relativity that people certainly do notice.

For instance, take the peculiar habit objects have, of shrinking when they move further away and growing large when they come closer.

I was once standing at the bus-stop, waiting for my particular vehicle to arrive. When I finally spotted it coming up the road, it was so small I could block it from view with just my little finger! I would scarcely have been able to fit a paper-clip inside, let alone my whole body.

Luckily, it grew as it approached, and eventually had enough room to accommodate myself, the driver, the conductor, and, indeed, all the other passengers as well.

The weird part is, this size-change depends on where you are. I saw some people board the bus at the previous stop, while it was still no bigger than a pendrive. I wouldn’t have thought of putting even a thumb inside, but the three passengers could fit themselves in quite comfortably. They were small, too.

But that’s only from my point of view. As the passengers saw it, they would have been the right size all along, and I would have been the one that was small.

Small, mind you. Not big, but small.

You’d have thought that if one saw the others small, the others would see the first one big, wouldn’t you? That makes sense. After all, how can the passengers and I both be smaller than each other?

The reason this happens is that our sizes are related to distance — and distance depends on your point of view.

There is no ‘true’ distance of an object. Anyone who speaks about distance has to answer the question: distance from what? Different people can be different distances away from the same object, so each will see it as coming to a different size.

Of course, we could all decide on a certain spot — a TV camera, say — and agree to measure distances from there. That would be useful in some cases — like if you’re shooting for a TV programme — but it’s still arbitrary and could well have been something else.

Interestingly, Special Relativity describes a similar thing happening, but with movement. You can never tell if an object is moving smoothly or standing still, unless you decide what it’s moving compared to.

A train could be moving at 50 kilometres an hour compared to the trees outside, 29.8 kilometres per second compared to the Sun, not at all compared to the passenger playing Temple Run on a smartphone, and only a little over 10 kilometres per hour compared to the vendor going “chai-aa, chai!” and selling tea. Neither of those speeds is more ‘true’ than the others: it all depends on your point of view.

Distance depends on your point of view, or “frame of reference” as they call it. But what about size?

I’ll always be closer to myself than to the passengers, no matter how close they come. But if that distance is small enough, it won’t make any difference (or rather, the difference won’t be noticed).

So maybe we can now answer the question: what is the ‘true’ size of an object?

Right now, I’m holding up a ruler to measure my cat. She appears to be barely an inch long, from head to tail. That’s somewhat surprising, because I’ve heard of newborn mice being bigger than that.

The reason, I realised, was that the ruler was closer to me than the cat. So it hadn’t shrunk as much, and ended up giving me a much smaller measurement than otherwise. That’s why most people, when measuring objects, put the ruler right up against the object so they’re almost touching. That way, the ruler and the object shrink by exactly the same amount.

No matter how far you are from the object and ruler, the measurement will always come to the same number of inches or centimetres.

But what is a centimetre? You can say a centimetre is the distance marked on a ruler. But how do you define it by length on a ruler, if the ruler’s length keeps changing? When lengths were first standardised, they defined a ‘metre’ as the length of one particular metal rod kept in Paris. But they perhaps got confused when their distance from Paris kept changing, because now a ‘metre’ is defined as the distance light travels through a vacuum in 1/299,792,458th of a second.

I like light. It’s the most reliable thing in the universe.

Other things may grow or shrink or speed up or slow down, depending on the situation. But light, no matter where you are, will always travel through a vacuum at exactly 299,792,458 metres per second. (That’s through a vacuum, mind you: if it’s going through glass or water or something it will slow down — but its speed is predictable as long as it’s travelling through nothing at all).

This gives us another convenient way to measure the length of a bus. You just have to measure how long it takes for light to go from one end to the other.

Of course, that’s a bit impractical when it comes to buses. Light travels so quick, you’d have to think faster than a fly. In fact, light moves so fast it could go round the Earth seven times in a single second.

Still, the speed of light is convenient for measuring larger lengths, like the distance to the Moon.

Speed is a tricky thing. It’s not just about the distance travelled, but about the distance travelled per second. That means it’s got to do with time — and time, as we all know, is relative.

For example, when I was standing to catch the bus, I had to wait there for a very long time. But from the perspective to my watch, the whole time I stood turned out to be merely five minutes.

“When you sit with a nice girl for two hours you think it’s only a minute, but when you sit on a hot stove for a minute you think it’s two hours. That’s relativity.”
—Albert Einstein

When Bill Bryson took a flight from the USA to Australia, he was intrigued by the fact that he had missed a day. He left Los Angeles on the 3rd of January, and arrived in Sydney fourteen hours later, on the 5th. It wasn’t that the 4th of January was very short or went by very fast. It just didn’t happen at all.

The reason for that has to do with timezones and the International Date Line. Luckily for Bill Bryson, he got compensated on the way back, when he left Sydney one day and arrived at Los Angeles on the previous one.

That wasn’t the case with Samoa, which once decided to change its timezone to the other side of the date-line. To do that, the island nation had to skip a whole Friday — as far as they were concerned, that particular day just didn’t exist.

Of course, this phenomenon is a bit different from Einstein’s relativity. It’s all artificial, and related to the fact that different people set their clocks to different times. They may not agree about the exact date Bill Bryson landed, but they’ll all agree that the flight took fourteen hours.

Or will they?

Well, not if they get very precise. Apparently, gravity speeds up time. Or is it inertia, the force that pushes you back when you go faster? Actually, they’re both the same thing; it just depends on your point of view. Whichever it is, the point is, more of it makes time go faster, and less makes it go slow.

Much less confusing than having to keep track of what country is where and what timezones they use and which side of the International Date Line they happen to be on.

Lots of people like to talk about Time and Relativity. You can find discussions on it all over the place. What’s not so well-known is the relation between Special Relativity and length.

You see, when things are moving faster, they also become shorter.

Now don’t ask who is moving and who becomes shorter and why doesn’t the other person become longer. It’s all relative, like me and the bus. If the bus goes past fast, I’ll see it very short with a very thin driver, while the driver will see me as being very thin.

Note that, with moving, the shrinking happens only in one direction. Things don’t become smaller, they just get squashed in the direction they’re going, like a photograph with the wrong aspect-ratio.

This squashing is important to light, because what if the conductor at the back shines a torch to the front of the bus? It’ll travel the length of the bus, 🚌, in a certain amount of seconds, ⌛.

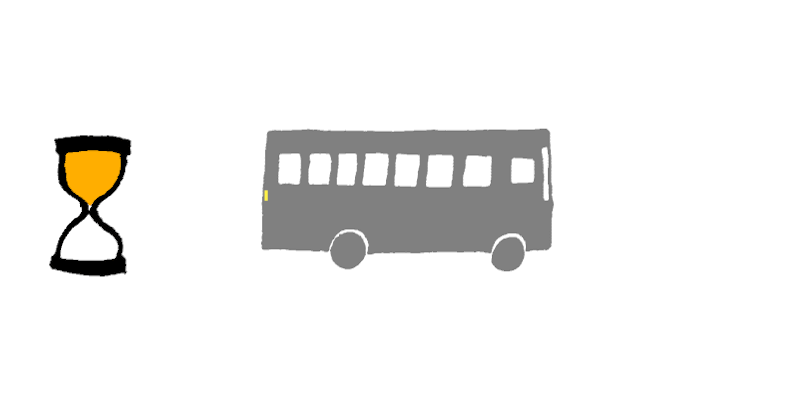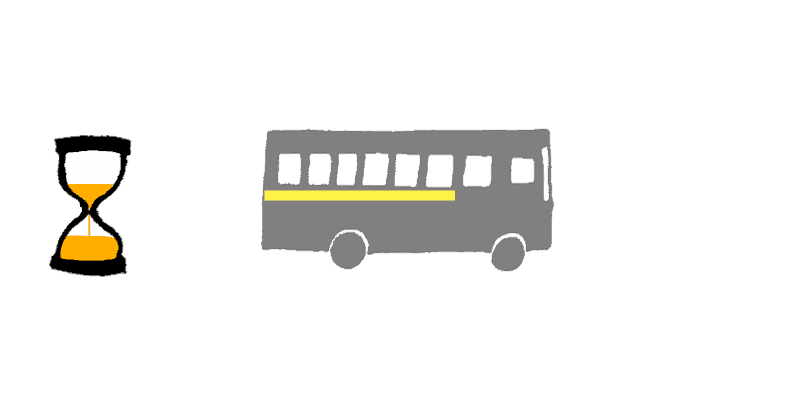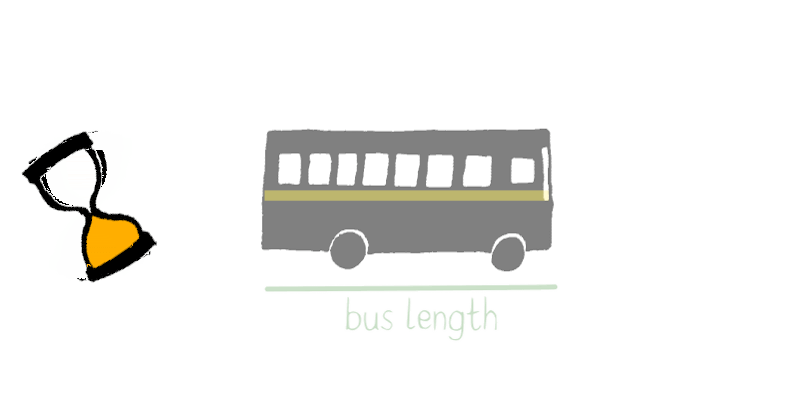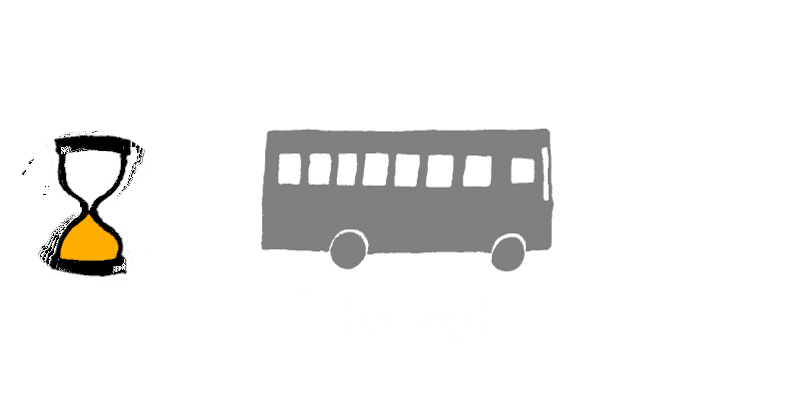
At least, according to people inside the bus.

But what about for me? The bus is moving. So I won’t just see the light go from the back to the front of the bus. I’ll see it go from where the back of the bus was at the beginning, to where the front ends up at the end.

That’s the length of the bus, 🚌, plus the distance it travelled, 🛣, all in the same old amount of time, ⌛! Is light travelling faster for me than it is for the bus-conductor?

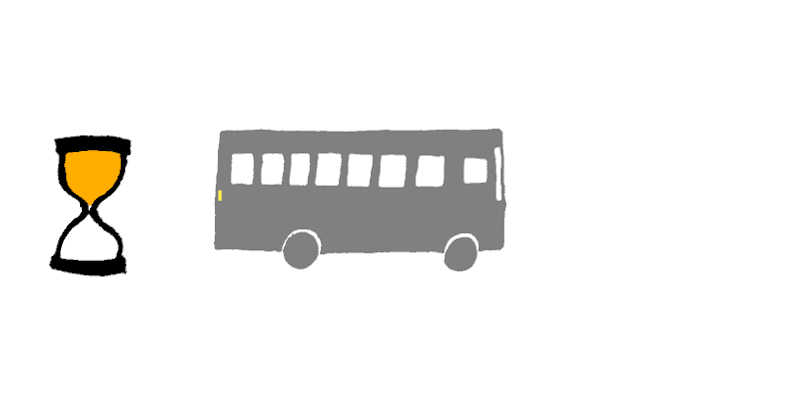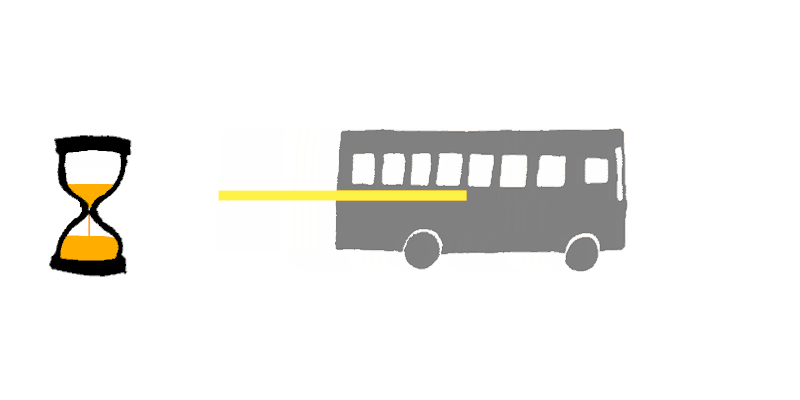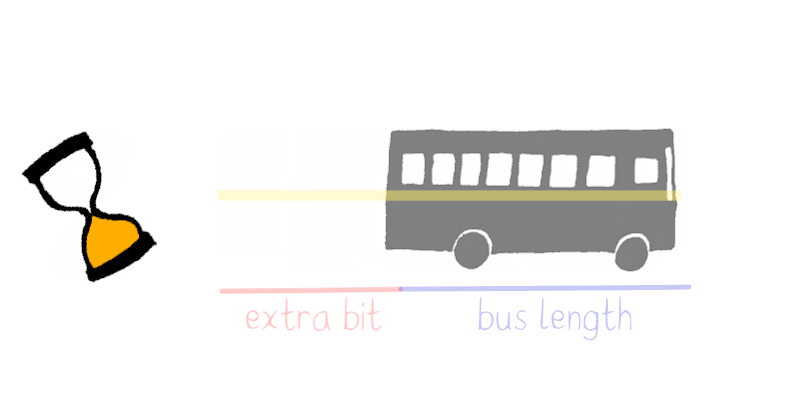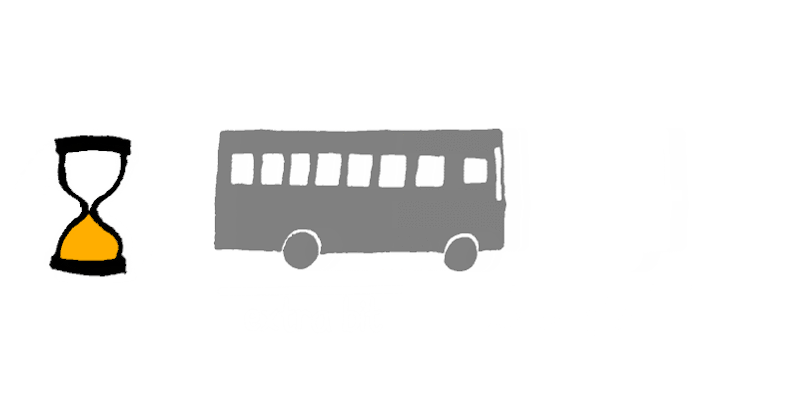
No, it’s not. The bus is moving, so “length of the bus, 🚌” is actually less for me than it is for the conductor. How much less? As it turns out, it’s just enough less to make up for the extra distance, 🛣, that I saw the bus travel.

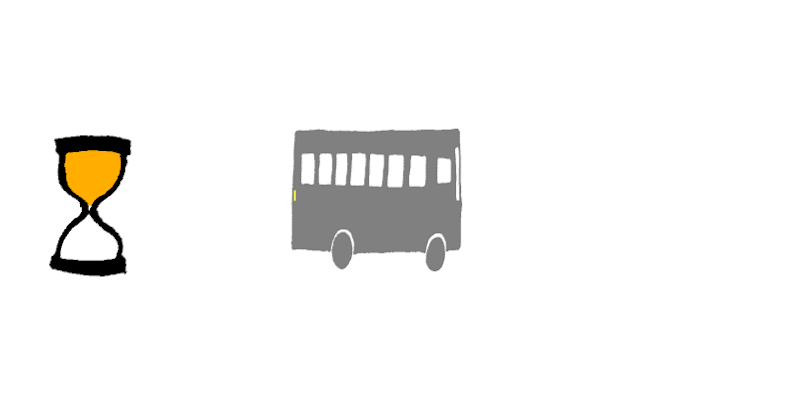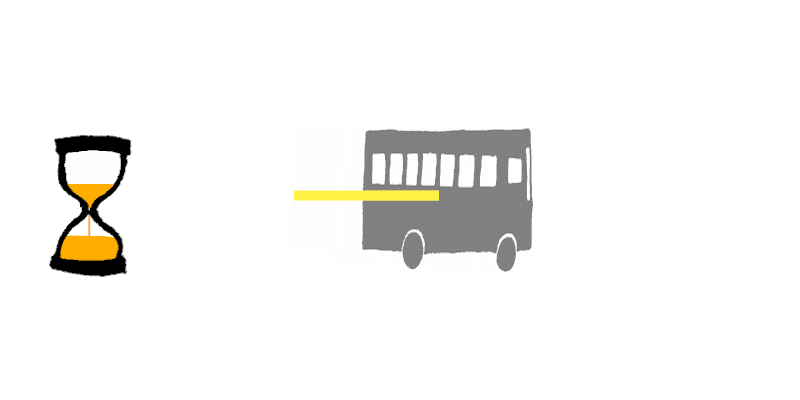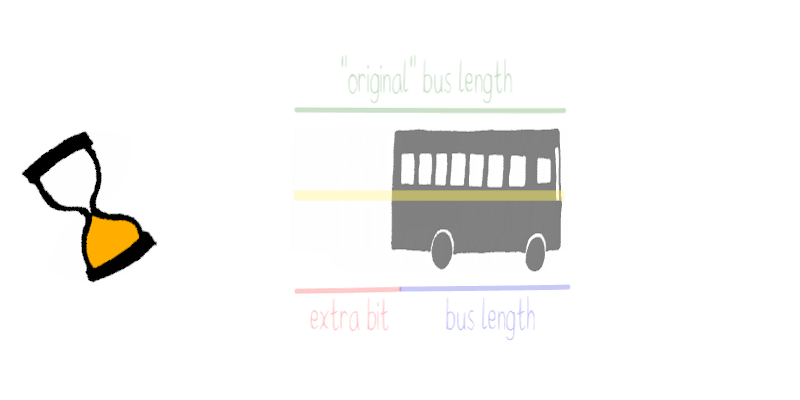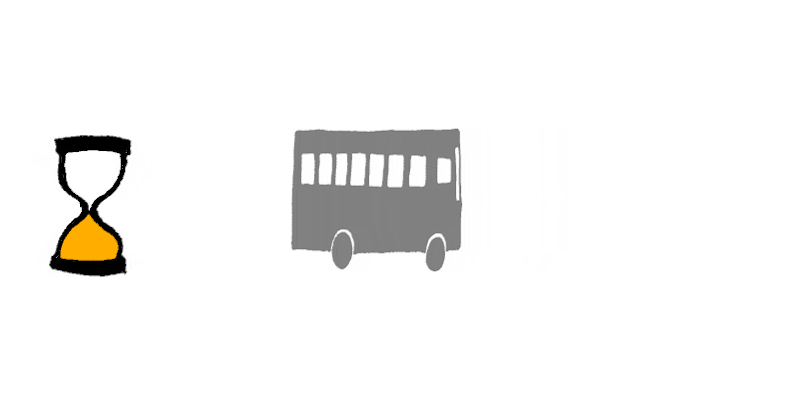
My distance 🚌+🛣 is the same as the conductor’s distance 🚌, so that means the distance light travelled in that time ⌛ is the same for both of us. Which means, so is the speed.

So that’s one neat trick light uses, to travel the same way for everyone.

It may take a bit of getting used to — but in the end, all you have to remember is that light in a vacuum always travels at the same speed. And the rest of relativity, I’m sure, is equally simple.

If you were to write it all down in a book, it would make for a very small book. (Relatively speaking, of course).

This piece was inspired by “Direction of the Road”, a short story by Ursula K. Le Guin.

Have something to say? At Snipette, we encourage questions, comments, corrections and clarifications — even if they are something that can be easily Googled! Or you can simply click on the ‘👏 clap’ button, to tell us how much you liked reading this.